Conic Sections - Hyperbola, Equation of Hyperbola, Conic Sections, Co-Ordinate Geometry

Hyperbola: When the ratio (defined in parabola and ellipse) is greater than 1, i.e., e > 1, then the conic is said to be hyperbola.

Since the equation of the hyperbola

  differs from that of the a b2, most of the results proved for the ellipse are true for the hyperbola, if we replace b² by - b² in their proofs. We therefore, give below the list of corresponding results applicable in case of hyperbola. 

(1)     Standard equation of hyperbola is

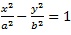
                   Where

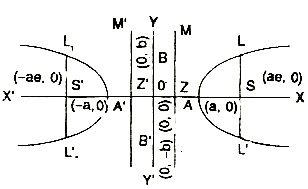
  In this case,
       

(i)      Foci are S (ae, 0) and S' (- ae, 0).

(ii)     Equations of directrix ZM ,and Z'M'

 

(iii)    Transverse axis AA' = 2a, conjugate axis BB' = 2b.

(iv)    Centre O (0, 0).

(v)     Length of latus rectum LL' = L1L1'

(vi)    The difference of focal distances from any point P(x1, y1) on hyperbola remains constant and is equal to the length of transverse axis. i.e.,

S'P - SP = (ex1 + a) - (ex1 - a) = 2a.
 

(2)     The equation of rectangular hyperbola x² - y² = a² = b² i.e., in standard form of hyperbola put a = b.

Hence e =

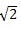
for rectangular hyperbola.

(3)     Parametric equations for hyperbola are x = a sec θ and y = b tan θ

(4)     Equation of tangent at any point

(x1, y1) on hyperbola is

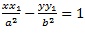
(5)     Equation of tangent in slope form is

y = mx ±

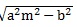
i.e., the line y = mx + c is a tangent to hyperbola,

if c = ±

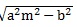
  and point of contact is

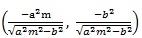
 (6)     the equation of normal at any point (x1, y1) on hyperbola is

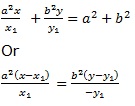
    (7)     Equation of chord of contact at point

(8)     Chord whose mid-point is (h, k) is

(9)     Chord joining 't1' and 't2' is x + yt1t2 - c(t1 + t2) = 0. 

(10)   tangent at t1 is x + yt12 - 2ct, = 0

 
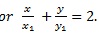
                   where x1 = ct1,

 

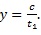
(11)   The length of chord cut off by hyperbola from the line y = mx + c is

           

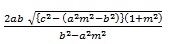
(12)   Equation of director circle is x² + y² = a² - b²
 

(13)   If Y = m1x and y = m₂x are the conjugate diameters of hyperbola, then

 
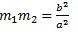
(14)   Conjugate Hyperbola: The hyperbola whose transverse and conjugate axes are respectively the conjugate and transverse axes of the hyperbola is called the conjugate hyperbola of the given hyperbola.

Thus is

= 1 be the given hyperbola, then

  

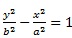
 conjugate hyperbola.

 

If e and e' are the eccentricities of these two hyperbolas, then

   

(15)   Equation ax² + 2hxy + by² + 2gx + 2fy + c = 0 represents a hyperbola, if Δ ¹ 0, h2 > ab and rectangular hyperbola if Δ ¹ 0, h2 > ab and a + b = 0.

 

Live Math Experts: Help with Hyperbola Assignments - Homework

Expertsmind.com offers help with Hyperbola assignment and homework in mathematics subject. Experts mind's mathexperts are highly qualified and experienced and they can solve your complex Hyperbola math problems within quick time. We offer email based assignment help -homework help service in all math topics including Hyperbola .

Math Online Tutoring: Hyperbola, Equation of Hyperbola

We at Expertsmind.com arrange instant online tutoring session in Hyperbola math topic. We provide latest technology based whiteboard where you can take session just like live classrooms. Math experts at expertsmind.com make clear concepts and theory in Hyperbola Math topic and provide you tricky approach to solve complex Hyperbola problems.